Archive:: Applied and Computational Mathematics:: Science Publishing Group

Volume 7, Issue 1, February 2018

A High Order Compact ADI Method for Solving 3D Unsteady Convection Diffusion Problems

Yongbin Ge, Fei Zhao, Jianying Wei

Issue: Volume 7, Issue 1, February 2018

Pages: 1-10

Received: 3 December 2017

Accepted: 13 December 2017

Published: 12 January 2018

DOI: 10.11648/j.acm.20180701.11

Downloads:

Views:

Abstract: In this paper, we develop a rational high order compact alternating direction implicit (RHOC ADI) method for solving the three dimensional (3D) unsteady convection diffusion equation. The present scheme, based on the idea of the fourth order rational compact finite difference operator for the spatial discretization and the Crank-Nicolson method for... Show More

Download PDF

View Online
A Stable and Convergent Finite Difference Scheme for 2D Incompressible Nonlinear Viscoelastic Fluid Dynamics Problem

Yanhua Cao, Zhendong Luo

Issue: Volume 7, Issue 1, February 2018

Pages: 11-18

Received: 4 December 2017

Accepted: 15 December 2017

Published: 12 January 2018

DOI: 10.11648/j.acm.20180701.12

Downloads:

Views:

Abstract: In this study, a stable and convergent finite difference (FD) scheme based on staggered meshes for two-dimensional (2D) incompressible nonlinear viscoelastic fluid dynamics problem including the velocity vector field and the pressure field as well as the deformation tensor matrix is established in order to find numerical solutions for the problem. ... Show More

Download PDF

View Online
A Reduced-Order Extrapolating Finite Difference Iterative Scheme for 2D Generalized Nonlinear Sine-Gordon Equation

Hong Xia, Fei Teng, Zhendong Luo

Issue: Volume 7, Issue 1, February 2018

Pages: 19-25

Received: 17 December 2017

Accepted: 2 January 2018

Published: 18 January 2018

DOI: 10.11648/j.acm.20180701.13

Downloads:

Views:

Abstract: In this study, a reduced-order extrapolating finite difference iterative (ROEFDI) scheme holding sufficiently high accuracy but containing very few degrees of freedom for the two-dimensional (2D) generalized nonlinear Sine-Gordon equation is built via the proper orthogonal decomposition. The stability and convergence of the ROEFDI solutions are ana... Show More

Download PDF

View Online
Topological Structure of Riesz Sequence Spaces

Merve Temizer Ersoy, Bilal Altay, Hasan Furkan

Issue: Volume 7, Issue 1, February 2018

Pages: 26-30

Received: 29 December 2017

Accepted: 12 January 2018

Published: 20 January 2018

DOI: 10.11648/j.acm.20180701.14

Downloads:

Views:

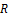
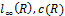
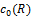
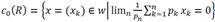
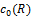
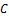
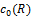
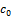
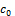
Abstract: In this paper, to be the Riesz matrix is symbolized by , it is defined the spaces and where for instance and computed its duals (α-dual, β-dual and γ-dual). Furthermore, it is investigated topological structure of and determined necessary and sufficient conditions for a matrix to map , or into or .

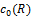
Download PDF

View Online